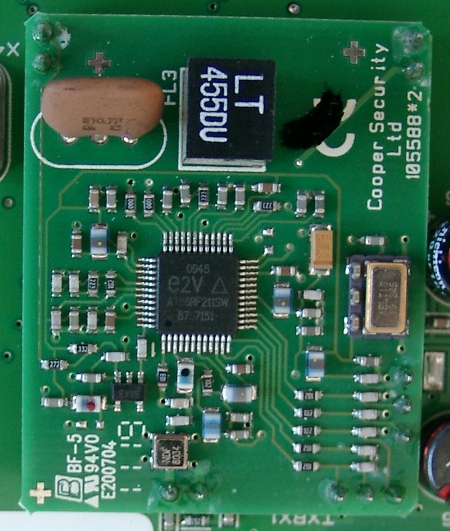
Reverse-engineering the ABUS Secvest Wireless Intruder Alarm System's Radio Protocol
May 10, 2012 at 01:00 AM | categories: reverse engineering, wirelessThis report documents an analysis of the wireless interface of an ABUS Secvest burglar alarm system. The analysis is based on passively received radio datagrams, which were reverse-engineered. Because the protocol offers no protection mechanism against attacks, the Secvest system cannot grant confidentiality, integrity, and authenticity of communication. This is a security weakness, especially if messages are transmitted via a shared and remotely accessible medium.
Until now there is no public review available in which the effective protection level of the Secvest system is examined. The weak link is the system’s wireless interface: ABUS doesn’t use encryption and message authentication and not even a simple rolling code, which is a common technique even for garage door openers. The lack of protection mechanisms allows an attacker to eavesdrop radio communication and to inject their own datagrams by spoofing addresses of components belonging to an alarm system.
Brute-forcing CRC parameters
February 10, 2012 at 11:20 AM | categories: tools, reverse engineeringRecently, I had to discover the parameters for a cycling redundancy check (CRC) calculation. Because it was the second time within six months that there was a need for determining a CRC polynomial, I implemented a brute-forcing tool. This tool reveals the (truncated) polynomial, the initial value, input and output reflection and a finally applied XOR-mask. Obviously, brute-forcing fails for large polynomials like for a CRC-32, because you have to try different polynomials and initial values. But for smaller CRCs this approach works well. Usually, you do not have truncated polynomials larger than 16, especially not in simple telegrams with some bytes.
What is a truncated polynomial? In general, a polynomial has a grade. E.g., CRC-16-CCITT has a polynomial of grade 16. This is x^16 + x^12+x^5+1. To describe the polynomial by its coefficients the leading digit is often skipped, e.g., 1.0001.0000.0010.0001 becomes 1.0000.0010.0001. Thus, the term truncated polynomial is used to indicate this truncation.
Let’s see how this tool works. In a first step, we generate some test data. Therefore, the tool generate-test-data might be used. It generates some random bit strings and calculates a CRC, which is appended to the string.
$ ./generate-test-data --final-xor 0 --messages 10 | tee testmessage_1 # width : 10 bits # CRC's offset : 49 # calc CRC for bit offsets : 0 .. 49 (not included) # final XOR : 0 # reflect in : true # reflect out : false # # truncated polynom : 189 (MSB not shown) # initial value : 149 0110110010000011101000011000110101111000000000100 1111111010 0001000000001100101100100110011011111100000110100 0101000101 1101011100111000110110110010111011110110101001001 0011100111 0100000011100011110000100111010001101100010010011 0110100011 0110110000010111010110011001010011100101110000101 1100000001 0110101000000111010111101101001000110010100111110 1011001001 1000101011110110010100001110001111101110000011000 1000010010 0110111010111000011100100111110101110101111011010 1001111001 1001101001111000010110001000011101110000111001001 0101110000 1101110110101001101001110010011101010001110000110 0000110111
Here, the bit string starts at offset 0 and ends at offset 49. Please note, that these are not the common eight bits per byte. I avoided this byte-centric view, because in low-level reverse engineering messages might be odd-formed, especially when analyzing radio transmission.
In a second step, these test messages are reformatted to fit the brute-forcing tool’s input format. Therefore, I wrote a script in Perl. It reads bit strings (“101001…”), hex strings (“0xdeadbeef…”) and comma-separated lists of hex values (“0x23, 0x42, 0x5”) and reformats them to either a hex or a bit string.
$ perl ../rewrite-as.pl bits testmessage_1 | tee testmessage_1.bits 01101100100000111010000110001101011110000000001001111111010 00010000000011001011001001100110111111000001101000101000101 11010111001110001101101100101110111101101010010010011100111 01000000111000111100001001110100011011000100100110110100011 01101100000101110101100110010100111001011100001011100000001 01101010000001110101111011010010001100101001111101011001001 10001010111101100101000011100011111011100000110001000010010 01101110101110000111001001111101011101011110110101001111001 10011010011110000101100010000111011100001110010010101110000 11011101101010011010011100100111010100011100001100000110111
Finally, we launch the CRC brute-forcing tool, which requires specifying several offsets: The tool needs to know, where the message’s CRC relevant portion starts and stops and where the checksum is placed. When reverse engineering a telegram format, one might determine the checksum part without problems. This is caused by the CRC algorithm’s property that it is a Galois type shift register, which produces pseudo-random numbers. But it loops not on its own. Instead the algorithm is fed with message bits. Thus, the checksum looks more random than the rest of the message.
$ ./bruteforce-crc --width 10 --start 0 --end 49 --offs-crc 49 \
--file testmessage_1.bits --probe-reflections true
number of threads : 4
width : 10 bits
CRC's offset : 49
calc CRC for bit offsets : 0 .. 49 (not included)
final XOR : 0
reflect in : false
reflect out : false
truncated polynom : from 0 to 1023 (MSB not shown)
initial value : from 0 to 1023
probe reflections : true
probe final xor : false
extracted crc 03fa
extracted crc 0145
extracted crc 00e7
extracted crc 01a3
extracted crc 0301
extracted crc 02c9
extracted crc 0212
extracted crc 0279
extracted crc 0170
extracted crc 0037
----------------------[ MATCH ]--------------------------------
Found a model for the CRC calculation:
Truncated polynom : 0xbd (189)
Initial value : 0x95 (149)
Final XOR : 0x0 (0)
Reflected input : false
Reflected output : false
Message offset : from bit 0 .. 49 (end not included)
The brute-forcing tool extracts the checksum field at the specified offset and compares the calculated CRCs with the extracted CRCs. If a CRC matches the extracted one, the tool continues with testing the other messages. And finally, if a CRC parameter set satisfies all messages a CRC model is found.
If you have to reverse engineer an undocumented bus-protocol or some radio messages and don’t want to reconstruct the CRC parameters by putting on one's thinking cap, you might need such a tool, too. Therefore, I published the code on github.com.
Further readings:
DARPA Shredder Challenge 2011
December 09, 2011 at 12:35 PM | categories: aktenvernichtungIn diesem Jahr veranstaltete die Defense Advanced Research Projects Agency (DARPA) ein Puzzeln der besonderen Art. Teilnehmer sollten geschredderte Dokumente wieder zusammensetzen. Nicht verwunderlich ist der Hintergrund:
"Today's troops often confiscate the remnants of destroyed documents in war zones, but reconstructing them is a daunting task. [...]The goal was to identify and assess potential capabilities that could be used by our warfighters operating in war zones, but might also create vulnerabilities to sensitive information that is protected through our own shredding practices throughout the U.S. national security community."
Die Formulierung klingt so, als wäre dieses Thema erst jetzt für die Sicherheitscommunity von Interesse. Dem ist natürlich nicht so, denn es gab Anlass genug, über geeignete Aktenvernichtung nachzudenken und Methoden zur automatischen Rekonstruktionzu entwickeln. Unabhänig davon ist es eine interessante Herausforderung aus den Bereichen Informationssicherheit, Bildverarbeitung und Methoden der Künstlichen Intelligenz.

Der Wettbewerb bestand aus fünf Runden mit teilweise mehreren Dokumentenseiten, Grafiken, z.T. liniertem und kariertem Papier und rundenverschiedenen Handschriften. Die Seiten wurden einzeln mit einem Cross-Cut-Shredder zerstört und mit 400 dpi eingescannt. In den ersten zwei Runden waren die Papierschnipsel größer und entsprechen etwa der Sicherheitsstufe 3 (nach DIN-Norm) für vertrauliches Schriftgut. Bei den letzten Runden waren die Schnipsel kleiner und entsprechen vom Flächeninhalt grob der Sicherheitsstufe 4 für geheimes Schriftgut.
Einige Teilnehmer haben ihre Ansätze dokumentiert und teilweise auch Programmcode veröffentlicht:
- Digital video and multimedia lab der Columbia University
- Deshredding-Tool
- You should probably start burning your mail: What I learned from the DARPA Shredder Challenge.
Update: Das Team "All Your Shreds Are Belong to U.S." machte sich bei einem der schwierigen Puzzels kleine gelbe Punkte zunutze, die von vielen Farblaserdruckern auf das Papier gebracht werden. Diese Punkte dienen dem Nachvollziehen von Fälschungen. Hier ermöglichten diese Punkte das Zusammensetzen der Schnipsel zu einem Dokument. Siehe auch Investigating Machine Identification Code Technology in Color Laser Printers.
Radare and the GCHQ's brainteaser
December 02, 2011 at 10:15 PM | categories: tools, reverse engineeringRecently I discovered the tool suite radare, which is a handy tool for analyzing snippets of binary code. You can just open the file containing shell code and disassemble it. Radare works with PE files and ELF binaries. Further, the software supports several machine types like ARM and Intel and allows analyzing code for Dalvik and Java virtual machines.
When I stumbled upon the GCHQs reverse engineering challenge, I noticed the 0x42424242, the 0x41414141 and the not that obvious “deadbeef”. I supposed that it is not a byte wise XOR with a “secret” key, at least not on the full message. The most specific hint for me to inspect the code with radare is the message’s first byte, which is a 0xeb. This is also the first byte on my hard disk and there it represents a jump to the boot loader.
The secret message is given as:
char bytes[] = {
0xeb, 0x04, 0xaf, 0xc2, 0xbf, 0xa3, 0x81, 0xec,
0x00, 0x01, 0x00, 0x00, 0x31, 0xc9, 0x88, 0x0c,
0x0c, 0xfe, 0xc1, 0x75, 0xf9, 0x31, 0xc0, 0xba,
0xef, 0xbe, 0xad, 0xde, 0x02, 0x04, 0x0c, 0x00,
0xd0, 0xc1, 0xca, 0x08, 0x8a, 0x1c, 0x0c, 0x8a,
0x3c, 0x04, 0x88, 0x1c, 0x04, 0x88, 0x3c, 0x0c,
0xfe, 0xc1, 0x75, 0xe8, 0xe9, 0x5c, 0x00, 0x00,
0x00, 0x89, 0xe3, 0x81, 0xc3, 0x04, 0x00, 0x00,
0x00, 0x5c, 0x58, 0x3d, 0x41, 0x41, 0x41, 0x41,
0x75, 0x43, 0x58, 0x3d, 0x42, 0x42, 0x42, 0x42,
0x75, 0x3b, 0x5a, 0x89, 0xd1, 0x89, 0xe6, 0x89,
0xdf, 0x29, 0xcf, 0xf3, 0xa4, 0x89, 0xde, 0x89,
0xd1, 0x89, 0xdf, 0x29, 0xcf, 0x31, 0xc0, 0x31,
0xdb, 0x31, 0xd2, 0xfe, 0xc0, 0x02, 0x1c, 0x06,
0x8a, 0x14, 0x06, 0x8a, 0x34, 0x1e, 0x88, 0x34,
0x06, 0x88, 0x14, 0x1e, 0x00, 0xf2, 0x30, 0xf6,
0x8a, 0x1c, 0x16, 0x8a, 0x17, 0x30, 0xda, 0x88,
0x17, 0x47, 0x49, 0x75, 0xde, 0x31, 0xdb, 0x89,
0xd8, 0xfe, 0xc0, 0xcd, 0x80, 0x90, 0x90, 0xe8,
0x9d, 0xff, 0xff, 0xff, 0x41, 0x41, 0x41, 0x41
};
I just tried to decode it with radare and here it is:
[0x00000000]> eval asm.arch=intel32
[0x00000000]> pd
.=< 0x00000000, 0 cursor: eb04 jmp 0x6 ; 1 = 0x00000006
| 0x00000002 0 af scasd
| 0x00000003 0 c2bfa3 ret 0xa3bf
| 0x00000003 0 ; ------------------------------------
`-> 0x00000006 256_ 81ec00010000 sub esp, 0x100
0x0000000c, 256 31c9 xor ecx, ecx
.--> 0x0000000e 256 880c0c mov [esp+ecx], cl
| 0x00000011 256 fec1 inc cl
`==< 0x00000013 256 75f9 jnz 0xe ; 2 = 0x0000000e
0x00000015 256 31c0 xor eax, eax
0x00000017 256 baefbeadde mov edx, 0xdeadbeef ; (0xffffffffdeadbeef)
.---> 0x0000001c, 256 02040c add al, [esp+ecx]
| 0x0000001f 256 00d0 add al, dl
| 0x00000021 256 c1ca08 ror edx, 0x8
| 0x00000024, 256 8a1c0c mov bl, [esp+ecx]
| 0x00000027 256 8a3c04 mov bh, [esp+eax]
| 0x0000002a 256 881c04 mov [esp+eax], bl
| 0x0000002d 256 883c0c mov [esp+ecx], bh
| 0x00000030, 256 fec1 inc cl
`===< 0x00000032 256 75e8 jnz 0x1c ; 3 = 0x0000001c
.====< 0x00000034, 256 e95c000000 jmp 0x95 ; 4 = 0x00000095
| 0x00000039 256 89e3 mov ebx, esp
| 0x0000003b 256 81c304000000 add ebx, 0x4
| 0x00000041 248_ 5c pop esp
| 0x00000042 256_ 58 pop eax ; cursor+0x8
| 0x00000043 256 3d41414141 cmp eax, 0x41414141
.=====< 0x00000048, 256 7543 jnz 0x8d ; 5 = 0x0000008d
|| 0x0000004a 264_ 58 pop eax ; cursor+0x8
|| 0x0000004b 264 3d42424242 cmp eax, 0x42424242
.======< 0x00000050, 264 753b jnz 0x8d ; 6 = 0x0000008d
||| 0x00000052 256_ 5a pop edx
||| 0x00000053 256 89d1 mov ecx, edx
||| 0x00000055 256 89e6 mov esi, esp
||| 0x00000057 256 89df mov edi, ebx
||| 0x00000059 256 29cf sub edi, ecx
||| 0x0000005b 256 f3a4 rep movsb
||| 0x0000005d 256 89de mov esi, ebx
||| 0x0000005f 256 89d1 mov ecx, edx
||| 0x00000061 256 89df mov edi, ebx
||| 0x00000063 256 29cf sub edi, ecx
||| 0x00000065 256 31c0 xor eax, eax
||| 0x00000067 256 31db xor ebx, ebx
||| 0x00000069 256 31d2 xor edx, edx
.-------> 0x0000006b 256 fec0 inc al
|||| 0x0000006d 256 021c06 add bl, [esi+eax]
|||| 0x00000070, 256 8a1406 mov dl, [esi+eax]
|||| 0x00000073 256 8a341e mov dh, [esi+ebx]
|||| 0x00000076 256 883406 mov [esi+eax], dh
|||| 0x00000079 256 88141e mov [esi+ebx], dl
|||| 0x0000007c, 256 00f2 add dl, dh
|||| 0x0000007e 256 30f6 xor dh, dh
|||| 0x00000080, 256 8a1c16 mov bl, [esi+edx]
|||| 0x00000083 256 8a17 mov dl, [edi]
|||| 0x00000085 256 30da xor dl, bl
|||| 0x00000087 256 8817 mov [edi], dl
|||| 0x00000089 256 47 inc edi
|||| 0x0000008a 256 49 dec ecx
`=======< 0x0000008b 256 75de jnz 0x6b ; 7 = 0x0000006b
``-----> 0x0000008d 256 31db xor ebx, ebx
| 0x0000008f 256 89d8 mov eax, ebx
| 0x00000091 256 fec0 inc al
| 0x00000093 256 cd80 int 0x80
`----> 0x00000095 256 90 nop
0x00000096 256 90 nop
0x00000097 256 e89dffffff call 0x39 ; 8 = 0x00000039
0x0000009c, 256 41 inc ecx
0x0000009d 256 41 inc ecx
0x0000009e 256 41 inc ecx
0x0000009f 256 41 inc ecx
[0x00000000]>
Update: Heise has details on solving the whole puzzle.
Hello World
December 02, 2011 at 03:25 PM | categories: generalWelcome to my new blog. I will use this blog for IT security related postings, but it is not limited to. Please do not expect frequent updates.
IT security testing services
Over at Pentagrid, we provide pentesting services.
Latest blog posts
- Penetration testing services
- Simple server and service availability testing script with SMS notification
- Konferenz des DKI zu IT-Sicherheit im Krankenhaus
- Forensik-Challenge des Bundesnachrichtendienstes
- Fensterbrief - a python script for working with letters based on LaTeX and Markdown
- Traveling like a pro – an eight step guide to master business trips in Europe and to avoid common pitfalls
- Update
- JointSpace Server Directory Traversal Vulnerability on a Philips 6000 Series Smart LED TV
- Stack-based Buffer Overflow in the VPN Software tinc for Authenticated Peers
- Erster Platz beim Univention Absolventenpreis 2012
Categories
- advisory (2)
- aktenvernichtung (1)
- bnd (1)
- degate (1)
- forensik (1)
- general (5)
- latex (1)
- reverse engineering (4)
- tools (4)
- wireless (1)
